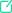题目信息
For each positive integer k, let a k =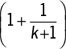 
. Is the product a1a2 … an an integer?
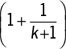. Is the product a1a2 … an an integer?
A:Statement (1) ALONE is sufficient, but statement (2) alone is not sufficient.
B:Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
C:BOTH statements TOGETHER are sufficient, but NEITHER statement ALONE is sufficient.
D:EACH statement ALONE is sufficient.
E:Statements (1) and (2) TOGETHER are NOT sufficient.
参考答案及共享解析
共享解析来源为网络权威资源、GMAT高分考生等； 如有疑问，欢迎在评论区提问与讨论
本题耗时：
已选答案：
正确答案：
B:Statement (2) ALONE is sufficient, but statement (1) alone is not sufficient.
Algebra Series and sequences
Since
, it follows that the product can be written as 
. Therefore, the product a1a2 … an is an integer if and only if 
is an integer, or if and only if n is an even integer.
If n = 2, then n + 1 = 3 is a multiple of 3 and the product is
, which is an integer. However, if n = 5, then n + 1 = 6 is a multiple of 3 and the product is 
, which is not an integer; NOT sufficient.
If n is a multiple of 2, then by the remarks above it follows that the product is an integer; SUFFICIENT.
The correct answer is B;statement 2 alone is sufficient.
Since
, it follows that the product can be written as . Therefore, the product a1a2 … an is an integer if and only if is an integer, or if and only if n is an even integer.If n = 2, then n + 1 = 3 is a multiple of 3 and the product is
, which is an integer. However, if n = 5, then n + 1 = 6 is a multiple of 3 and the product is , which is not an integer; NOT sufficient.
If n is a multiple of 2, then by the remarks above it follows that the product is an integer; SUFFICIENT.The correct answer is B;statement 2 alone is sufficient.
加入收藏
在线答疑
题目来源
